A Visual Appreciation For Black-Scholes Delta

I was messing with the Black-Scholes equation (as one does for fun) and happened on another way to visually understand it.

A prerequisite for appreciating this angle is to be familiar with Black-Scholes in the first place. If you aren’t and would like an intuitive understanding of the equation check out:

⚡The Intuition Behind The Black Scholes Equation (Moontower)

This is a review of what you need from that post but if it’s still foggy you can go back to the whole post.

This is the B-S equation:

If you can replicate the cash flow of an asset with a strategy then the price of the asset should equal the cost of executing the strategy.

• The left-side of the equation (ie the call option price) is the asset
• The right-side of the equation is the strategy

Construct a long/short portfolio: Short the call (the left-side)Long the strategy (the right-side)

Strategy – call = 0 profit

The call price must equal the p/l of the strategy for there to be no arbitrage.

The right-side of the equation is the strategy that replicates a long call option. (To offset the actual call we are short)

Let’s break this down step-by-step:

• That strategy is a portfolio
• The value of that portfolio at expiration discounted to present value must equal the value of the call option today
• The portfolio has 2 components:
  • Shares: We need long shares of the underlying stock
  • Cash: We will need a loan to finance those shares (An important idea in derivates pricing via arbitrage pricing is that we assume the strategy is “self-financing”. That means you don’t need money to start. If you respond with “But I do have some money to start”, the self-financing paradigm is already taking care of the opportunity cost using the RFR. The computation remains valid.)

How much cash and shares do we need?

1. Share quantity in the portfolio
   
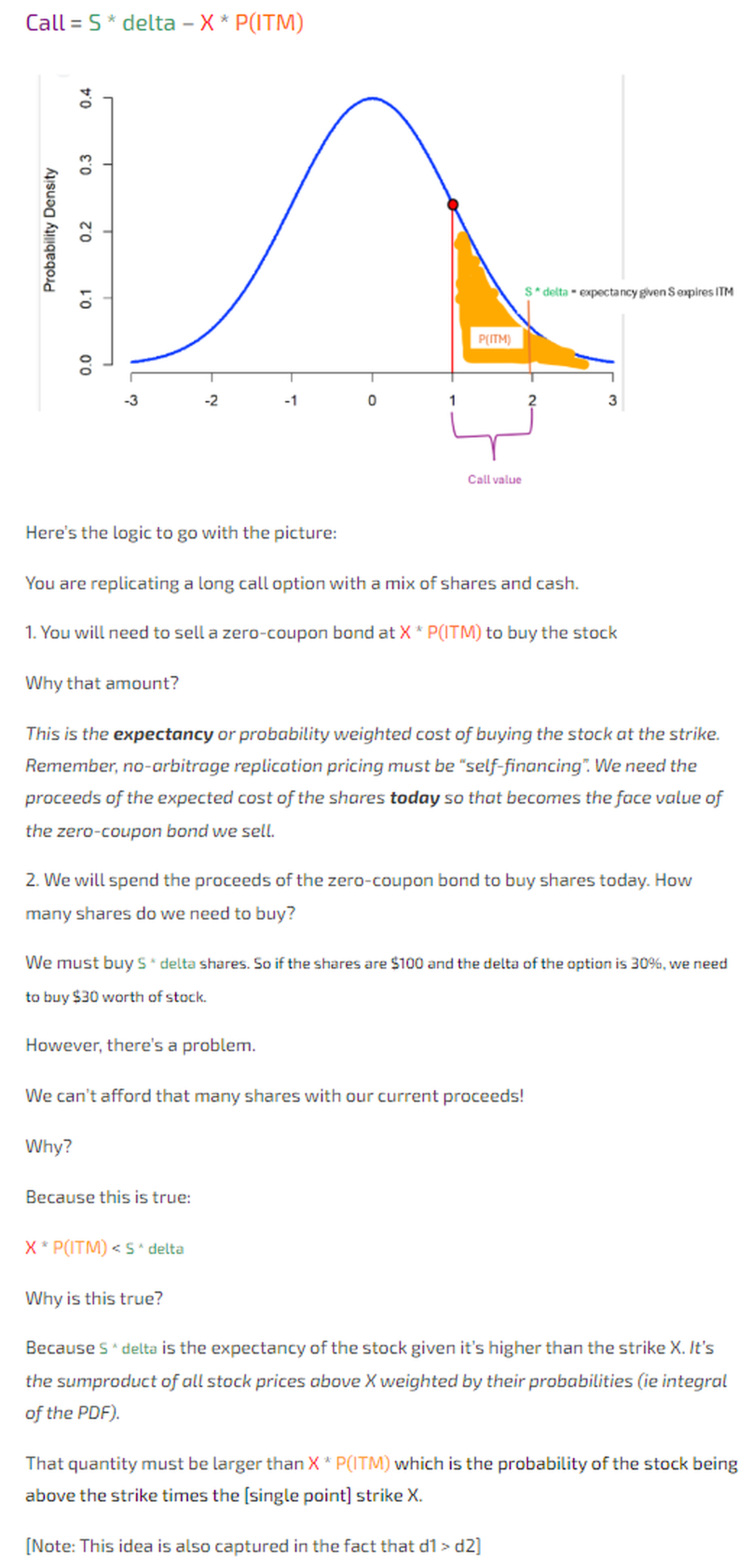
   The amount of stock you need in this replicating portfolio is weighted by the expected value of the strike being in the money. Notice we say “expected value” which is not just probability but probability x payoff. The phrase expected value of the shares going in the money is what determines the delta or hedge ratio of the option.Delta = N(d1)
   
   Share quantity =   S*N(d1)
   
2. Cash quantity in the portfolio
   
   We need cash to finance the purchase of those shares. If we are short the call and it goes in the money we know we will receive the strike price at expiration because the long option holder will exercise the call and we will sell shares to them. If the shares were 100% to be in-the-money then we know we would receive the strike price at expiration. For example, if you sold a call option struck at $125 and it was 100% to be in-the-money, you are certain to sell the stock at $125 and receive that much cash at that future date. Of course, the option is not 100% to be in the money. So we discount the strike in 2 ways:

• By the probability that it will be in the money
• By the risk-free rate, to get it in present value terms
  
  We can now say, on average, you will receive the present value of the strike weighted by its probability of being in the money.

Probability of strike being in-the-money = N(d2)

Again, this is just expected value logic. We weight the present value of the strike by its probability of being in the money.

Cash quantity = PV(strike) * N(d2)

This ends the review. The next section is new material. If this is still foggy zoom in on this part of the B-S primer: Animating The Equation

Visually Representing These Quantities On The Distribution 

First, let’s ignore interest.

Remember:

N(d1) = delta

N(d2) = Probability stock (S) finishes > strike price (X)

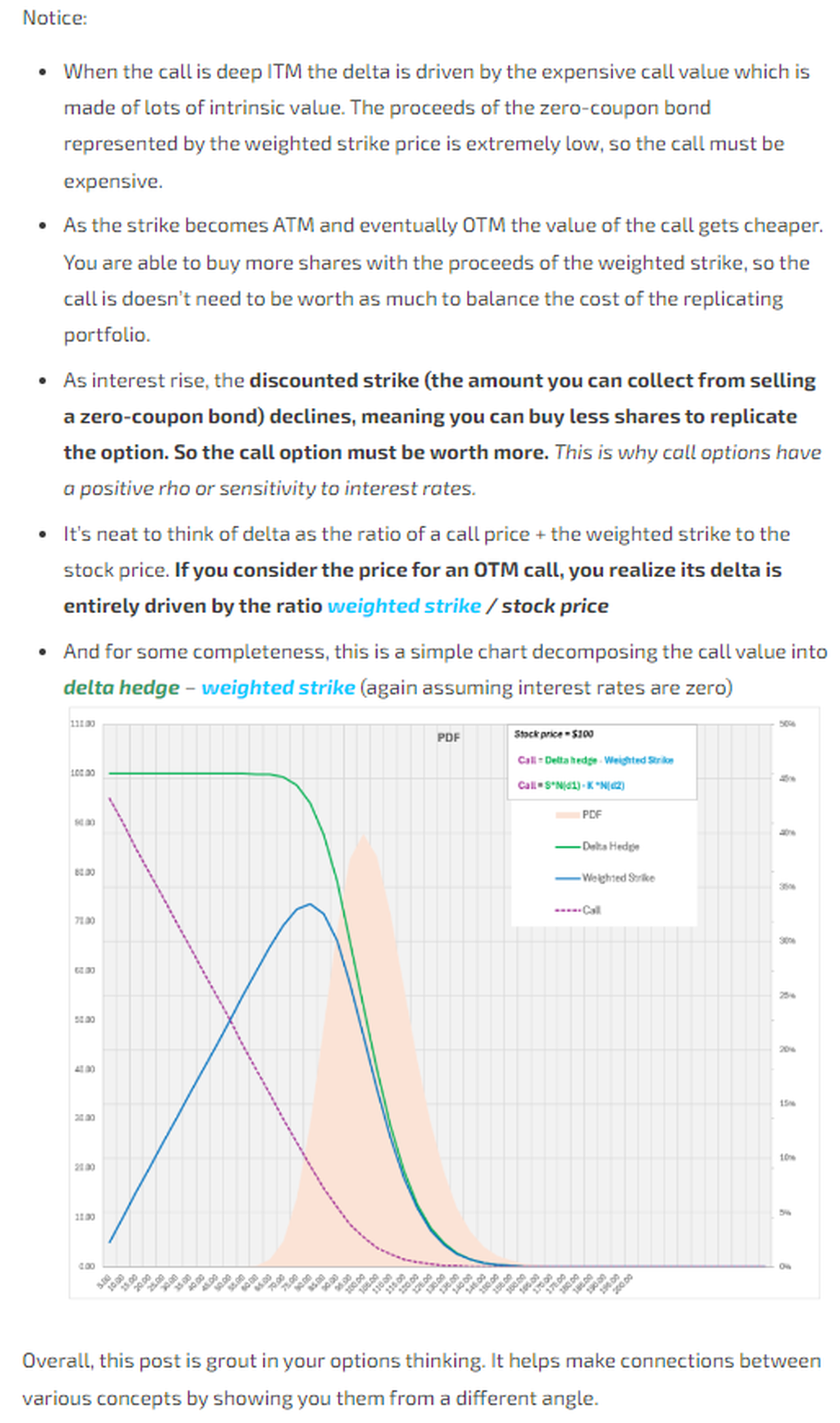
This shortfall in shares we can afford to buy with the proceeds of the zero-coupon bond sale is what the call option must be worth!

This is the essence of B-S. The call value is balancing price that equates the option to the cost of the replicating portfolio.

A Visual Decomposition Of Delta

We got this:

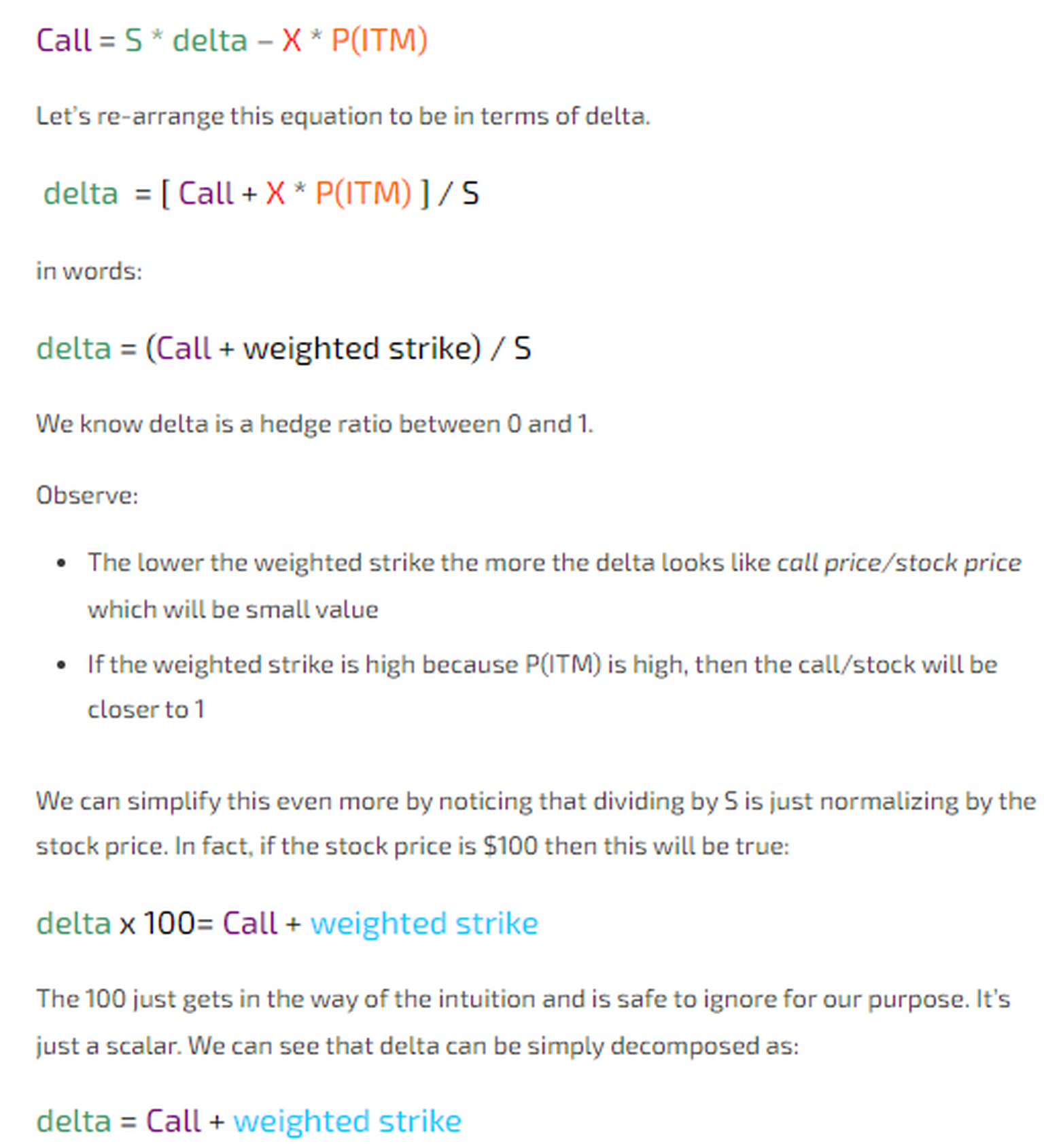
This decomposition is more satisfying visually. But before the grand reveal let’s just be thorough and validate that this calculation of delta matches what my B-S calculator says (it does).

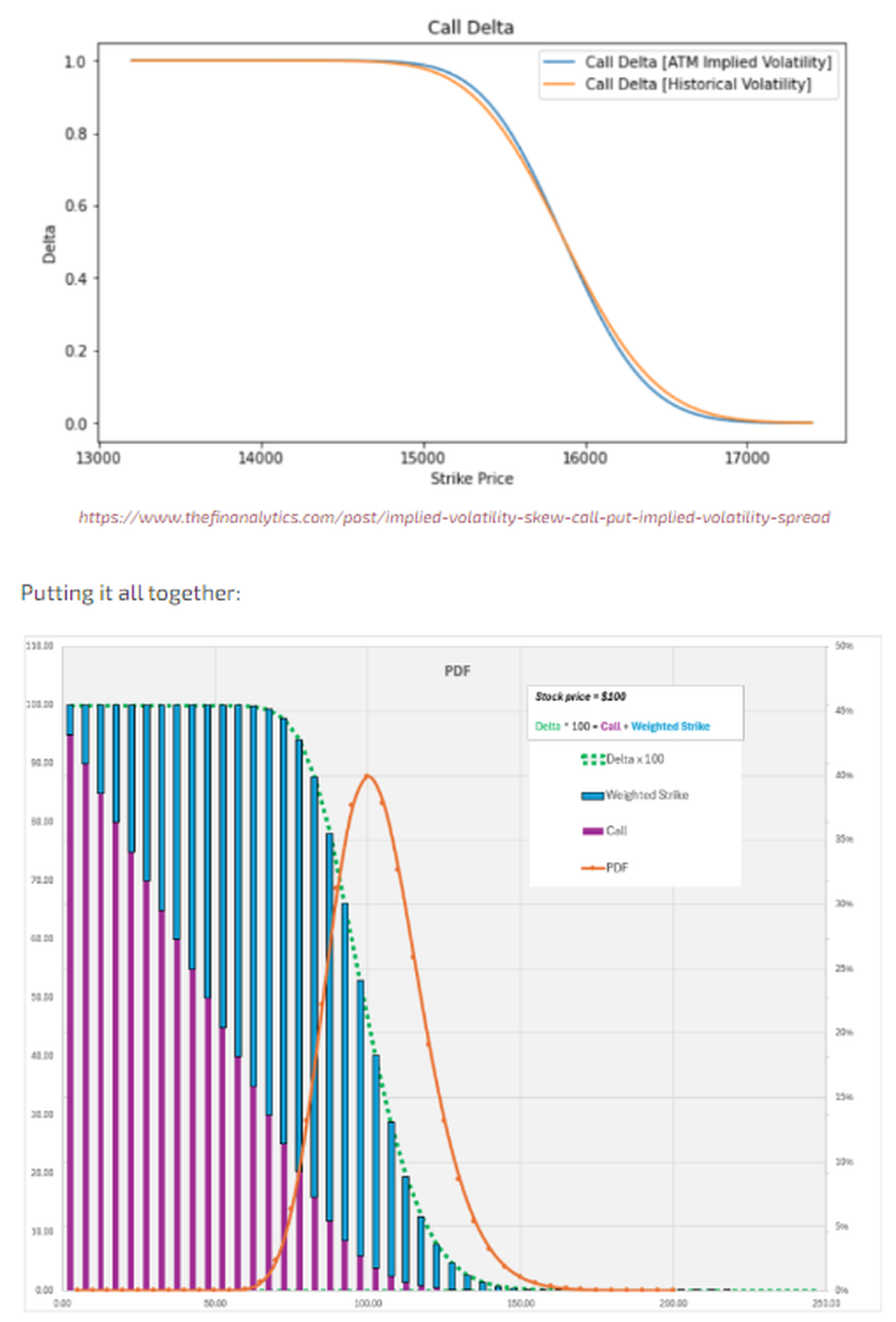
Let’s also remember what the chart of call delta by strike looks like: